It is important to be able to write proper electron configurations. The ordering of electrons in orbitals leads to specific properties for the given atom.
The periodic table was originally organized by the properties of the elements. Now we can see that it is in fact organized by the electronic structure of the elements. It is the structure of the electrons in these atoms from which the properties are derived. Elements have similar chemical properties as a result of having similar configurations of their valence electrons.
Atoms with multiple electrons are a complex subject. This is due to the fact that the wavefunction for an atom with more than one electrons is a function of the positions of all of the electrons in the atom. The electrons cannot really be thought of as being indpendent from each other. They are not independent from each other at all. However, this is very very difficult to deal with. As a result, as chemists we try to simplify the problem in a way that we can wrap our head around it. One very important simplfication that we make is that even though we cannot treat each electron separate, we do. This is known as the single electron approximation. And from it we get the very useful idea of "orbitals". We imagine that each electron has its own wavefunction and since wavefunction is an odd cumbersome word we instead use "orbital". To make things even more simple we assume these orbitals are essentially the same as the solutions we had for the hydrogen atom. The only difference being the nuclear charge. So we can use the same quantum numbers that we had for electrons in hydrogen to describe the electrons in a multi-electron atom.
However, it is important to recognize this is an approximation. In fact, whenever we try to discuss one electron in an atom versus another electron in an atom, it is an approximation. Thankfully it is a very good approximation!
To describe the electrons in multi-electron atoms, we use the same wavefunctions found in hydrogen. However, we now must add a new quantum number for electron spin, \( m_s \). There are two values which we call "spin up" or "spin down". These have values of +1/2 and -1/2. In general for atoms (and molecules) what is important is not the value plus or minus, but whether the electron is paired with another or not.
Another key idea is how many electrons we can place into the same orbital in a multi-electron atom. This idea derives from the Pauli Exclusion Principle. This principle tells us about specific properties that must hold for a wavefunction for a multi-electron system (technically the wave function must be anti-symmetric with respect to exchange of any two electrons). This results in a simple idea. No two electrons can have the same set of quantum numbers. This means that each orbital ( \( n, \ell , m_{\ell}\) ) can have only two electrons in it. One with each value of the spin ( \( m_s \) ).
Now we can simply place the electrons into the orbitals in order of increasing energy. Two electrons into each orbital. Unlike in the hydrogen atom, the energy now depends both on \( n \) and \( \ell \). Generally as the \( \ell \) quantum number increases the energy is slightly higher. As a result 2s and 2p are no longer identical in energy in atoms with more than one electron. Now 2p is slightly higher than 2s. Moreover, as the energy levels get closer and closer together the ordering of the energies with respect to \( n \) can change. However, in general the order stays the same. This is known as the Aufbau Principle.
This video looks at the quantum numbers for multi-electron atoms
Quantum Numbers for Multi-electron AtomsThe following video looks at the ground state electron configuration of lithium, Li. Note: there is a typo that state it is Be, when the video is about the electron configuration and corresponding quantum numbers for Li.
Electron ConfigurationsThe Aufbau Principle is the idea that we can find the electronic configuration of any element by simply "building up" from H and putting electrons into orbitals (wavefunctions) according to a rank in their energies. Aufbau means building up in German. Remember unlike in the hydrogen atom, the energy now depends both on \( n \) and \( \ell \). Generally as the \( \ell \) quantum number increases the energy is slightly higher. As a result 2s and 2p are no longer identical in energy in atoms with more than one electron. Now 2p is slightly higher than 2s. Moreover, as the energy levels get closer and closer together the ordering of the energies with respect to \( n \) can change. For example, we find that 3d is now higher in energy than 4s. Thankfully the general ranking of the energies of the subshells holds for most of the atoms. The order of the subshells is
\[1s<2s<2p<3s<3p<4s<3d<4p<5s<4d<5p\]
\[<6s<4f<5d<6p<7s<5f<6d<7p\]
We can determine the orbitals for the electrons in a multi-electron atoms by placing the electrons into subshells of ever increasing energy. We need to keep in mind that two electrons can go into each orbital. Thus a 3d subshell which has 5 d orbitals can "hold" 10 electrons. So we could place up to 10 electrons into the 3d subshell before needing to move on to the next highest energy subshell which is the 4p.
You should also get familiar with the periodic table as a "map" of the Aufbau filling order. As you "build up" the electrons in each atom, keep in mind just where you are on the periodic table. Here is a periodic table layout that is only showing the Aufbau filling order orbital sets. This is a far more useful diagram than any grid with diagonals marked on it.
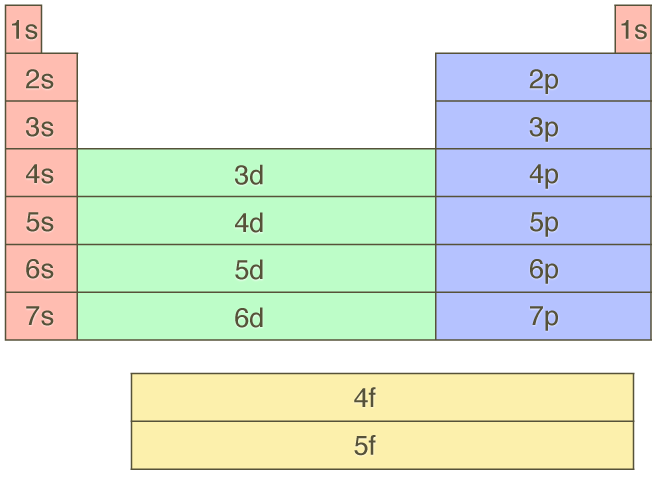
It is important to keep in mind that the Aufbau principle represents and approximate trend that holds in most cases. There are however exceptions to these rules. They will be covered in more detail later, but as a general idea the exception occur at places where a subshell can either be completely filled (or half-filled). Don't stress about the exceptions. Be very happy that this simple idea caputres 90% of the quantum mechanics of a very complicated problem. After this you can learn about the handful of exceptions and what they might tell us.
The Aufbau principle let's us build up an atoms electronic configuration by placing electrons into orbitals of every increasing energy. Hund's Rule tells us about how the electrons in an atom should be placed into degenerate orbitals. Technically Hund's Rules say that the ground state configuration should maximize the multiplicity of a configuration. What does that mean? The simple rule is that electrons should be placed into separate orbitals before going into the same orbital. This is because these best represent the configurations that are the lowest energy when we do the quantum mechanical calculation.
These pictures with up and down arrows for electron spin are a gross simplification of the realities of quantum mechanics. However, they do allow us to predict some measurable behavior for atoms such as the number of unpaired electrons. Thus Hund's rule has implications for predicting the properties of atoms as paired and unpaired electrons have distinct properties (in particular with interactions with magnetic fields).
Substances with unpaired electrons are called "paramagnetic" as they are attracted towards a magnetic field. Substances will all paired electrons are "diamagnetic" as they are repelled from a magnetic field.
Aufbau Principle and Hund's Rule\[1s^22s^22p^3\]
This does not denote anything about the spin of the electrons. In fact you need to be able to look at this configuration and know that the 2p electrons in nitrogen will be unpaired (each in a different orbitals) based on Hund's Rule.
This notation can get a bit tedious for configurations with lots of electrons. For example, the ground state configuration for Cl is
\[1s^22s^22p^63s^23p^5\]
To simplify this we often look only at the valence electrons and denote the core electrons with the corresponding noble gas structure. For Cl this would look like.
\[ [Ne]3s^23p^5\]
We typically look at the electron configuration for neutral atoms. However, we can also look at the configuration for atomic ions. These are atoms that are "missing" electrons, or have "extra" electrons. Here the idea is exactly the same. For cations (positive ions) you would simply remove the last electrons you placed into the subshell. For anions (negative ions) you would simply add more electrons (just as you would looking at an element with more electrons). Thus the configuration for a F- ion is
\[1s^22s^22p^6\]
Where one more electron has been placed into the 2p subshell for F-compared to F. You can see that F- has the same configuration that Ne would have. Since the electrons in F- and Ne have the same configuration we call these two species isoelectronic (same electrons). You will notice that many common atomic ions are isoelectronic with the noble gases as they have a very stable (low energy) electron configuration.
Below are several videos that show you how to systematically write electron configurations for the elements.
Dr. LaBrake shows the electron configurations of the elements of the first 2 periods (rows) of the periodic table. Hund's Rule is also illustrated.
Dr. LaBrake shows you how to use the periodic table to learn the Aufbau filling order of electrons in the atom.
Dr. Deborah Walker shows the power of the periodic table when doing electron configurations. She works examples for sulfur (S) and for telluriumm (Te).
Dr. LaBrake uses the Periodic Table to determine the electron configuration of iron. She shows both the shorthand notation and the long form.
Dr. LaBrake shows yet AGAIN, the power of using the Periodic Table to determine the electron configuration for bismuth (Bi).
As the Aufbau principle is a gross simplification of a quantum mechanical problem, we should be delighted that it works most of the time rather than being baffled by the fact that there are exceptions. Most of the exceptions to the rules occur for the d and f blocks where there is an added stability that comes from having either a half-filled or completely filled subshell. For example we find that the electrons is copper are better described by the configuration [Ar]4s13d10.
Exceptions to Filling Order