In chemistry we would like to quantify the amount of energy change associated with physical and chemical changes. This would be straight forward if we could simply measure the heat and work associated with any change. However, work is often difficult to quantify. Heat on the other hand is fairly straightforward to measure. Moreover, as we will see when we examine the second law of thermodynamics, heat flow is related to the entropy change for a process. As a result, we are very interested in the heat flow associated with chemical change.
In the lab, it is straightforward to quantify heat flow. It would be nice if there were a state function whose change was directly related to the amount heat that flows in a given process. As it turns out we can invent one: the enthlapy. Enthalpy is given the symbol H. In chemistry, the best way to think about the enthalpy is that it is very nearly equivalent to the "energy" of the system. Most importantly, the change in enthalpy for a process at constant pressure is exactly equal to the heat that flows between the system and the surroundings for that process. Thus, if we can quantify the heat flow at constant pressure, we can measure the change in the enthalpy of the system.
Because heat and work are not state functions, we cannot simply equate them individually to changes in state functions. However, under particular conditions this is possible.
For example, if we operate under conditions of constant volume, then the PV work is zero. In this case, the change in internal energy is simply equal to the heat flow.
\[q_v = \Delta U\]
We denote the conditions of constant volume with the subscript v.
However, in chemistry we almost always operate under conditions of constant pressure rather than constant volume. We would like a new state function that is equal to the heat flow at constant pressure. So we invent one and call it the enthalpy, H.
The heat at constant pressure is given by
\[q_p = \Delta U - w = \Delta U +P\Delta V\]
\[q_p = (U_f - U_i) +P(V_f - V_i) = (U_f + PV_f) - (U_i + PV_i)\]
Therefore, if we define a new state function, H that is defined as\[H = U +PV \]
We end up with the nice relation that the heat flow at constant pressure is equal to \(\Delta H\)\[q_p = H_f - H_i = \Delta H\]
So the enthalpy is nothing more than an invention of a new energy that is equivalent to the heat flow as measured at constant pressure. If the change is not at constant pressure then enthalpy is something, but it is not equal to the heat flow.
There are two other important chemical terms that we associate with enthalpy change. When a process lowers the enthalpy of the system, \(\Delta H < 0\), we call this process, exothermic. For an exothermic process at constant pressure, energy flows from the system to the surroundings in the form of heat. Combustion is an exothermic process that we are all familiar with. When a process increases the enthalpy of the system, \(\Delta H > 0\), we call this process endothermic. Ice melting is an endothermic process. Energy is absorbed by the system in the form of heat flow that leads to the solid converting to a liquid.
Let's examine the heat and enthalpy changes for a system undergoing physical change.
A good example that most people are familiar with is the heating of water. If we take a beaker filled with ice (solid water) and put in on a hot plate that has a temperature of 120 ° C we all know what will happen. First the ice will melt to liquid water. Then the water will increase in temperature. Then finally the water will boil. During this entire process the temperature of the hot plate will be higher than the temperature of the beaker of water. Thus, during this entire process energy will flow in the form of heat from the hot plate into the water. We would likely describe the water as the system and the hot plate as a constant temperature surrounding.
For this process, we are interested in the amount of heat that flows from surroundings into the system. Remember, heat can be tricky. When there is no chemistry or phase transitions, then energy flowing into a system in the form of heat will lead to a temperature change. However, when there is chemistry or a phase transition, then energy will flow in and the temperature can stay constant. Why doesn't the temperature go up? The energy coming in results in higher potential energy not higher kinetic energy. Breaking up the IMF between the molecules leads to a high potential energy.
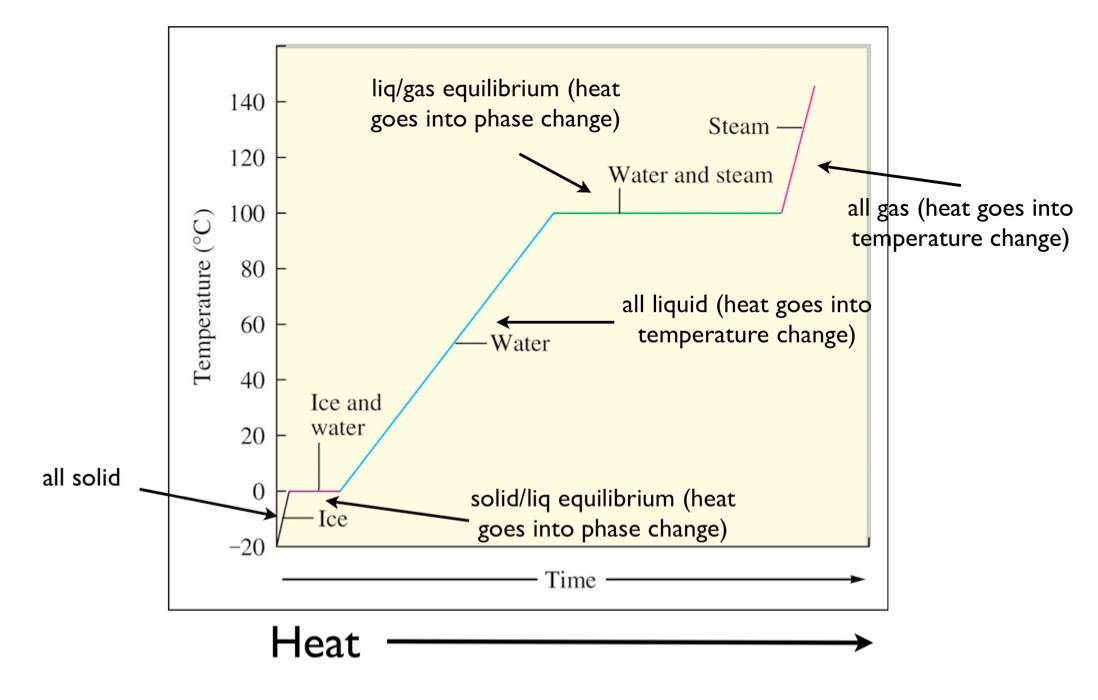
This can be easily seen in a heating curve that plots the temperature of a system as a function of the heat flow into the system. Initially the system is a solid, then it has a melting transition, then it is a liquid, then has a vaporization transition, and then it is a gas.
The diagram below shows the heating curve for water. The temperature of the system is plotted as function of time. Heat is flowing at a constant rate, thus time can be interpreted as heat.
Initially, the system is solid water at temperature of -20 °C. As the heat flows in, the temperature of the ice increases. The slope of this line is the heat capacity of solid water. Since this is at constant pressure then \(q = \Delta H = mC\Delta T\) where q is the heat, m is the mass, C is the specific heat capacity, and \(\Delta T\) the change in the temperature. As this graph is a plot of T vs q, the slope is actually 1/mC.
Next the solid melts. During this time the temperature is constant at 0 °C. Heat flows into the system, but the temperature does not change. At the start of this transition all of the water is solid. As heat flows into the system, the solid begins to melt into a liquid but the temperature stays constant. That is because the energy that is flowing into the system as heat is going into the potential energy of overcoming the intermolecular forces holding the water in a solid lattice. The length of the line at 0 °C is the amount of heat required to melt all of the solid into a liquid. This heat is called the enthalpy of fusion. Fusion is a specific scientific term for melting. You could call it the enthalpy of melting but instead it is called the enthalpy of fusion. The enthalpy of fusion is denoted as \(\Delta H_{fusion}\) and it typically is given in units of kJ mol-1. Thus the actual length of line will be \({n \times \Delta H_{fus}}\) since you'll need to multiply by the number of moles of solid to get the total heat of the phase transition.
Once all of the solid has been converted to liquid the temperature increases with heat flow. The slope of this line is again related to the heat capacity. However, this time it is the heat capacity of the liquid. Once the temperature reaches the boiling point of 100 °C the temperature is again constant as the liquid is converted to gas. The heat required to convert all the liquid to a gas is called the enthalpy of vaporization, \(\Delta H_{vaporization}\). You can see the heat required to vaporize water is significantly more than the heat required to melt water. This is true for most substances. Fusion involves simply breaking up the IMF to allow for translational motion of the molecules; vaporization requires essentially completely overcoming the IMF to move the molecules out of the condensed phase. Once all the liquid is converted to a gas, then the temperature increases with a slope related to the heat capacity of the gas.
The enthalpy associated with any phase change can be experimentally quantified and tabulated. Each particular phase change has an associated heat and is given a particular name.
For example, the enthalpy change for melting is defined as the enthalpy change for changing from a solid to a liquid at the melting temperature. For water this would be
\[ \rm H_2O(s, 0^{\circ}C) \rightarrow H_2O(l, 0^{\circ}C) \]
The process of going from solid to liquid is referred to a "fusion". The enthalpy for this change is \(\Delta H_{fusion}\). This a change in enthalpy,
\[\Delta H_{fusion} = H_f - H_i = H_{liquid} - H_{solid}\]
Because of this, the enthalpy for the opposite process, freezing, will be exactly equal but opposition in sign.
\[\Delta H_{freezing} = H_{solid} - H_{liquid} = -\Delta H_{fusion}\].
This means that only one value is ever tabulated. Typically it is the endothermic process. Thus, for solid/liquids the fusion value is tabulated. For liquids/gases you will find tabulated data for \(\Delta H_{vaporization}\). Again for the opposition process (gas to a liquid) the enthalpy is equal by opposite in sign.
\[\Delta H_{vaporization} = -\Delta H_{condensation}\]
.The same follows for the solid/gas phase transitions of sublimation (solid to gas) and deposition (gas to solid).
\[\Delta H_{sublimation} = -\Delta H_{deposition}\]
.For a chemical reaction, we often look at how much heat is associated with that particular chemical change. Any chemical change is associated with a balanced equation for that reaction. For example, if we examine the heat associated with reacting methane with oxygen gas to make carbon dioxide and water
\[\rm CH_4(g) + 2O_2(g) \rightarrow CO_2(g) + 2H_2O(g)\]
The heat for a reaction is given the symbol \(\Delta H_{\rm rxn}^\circ \). It has units of energy per mole reaction (rxn). Per mole reaction refers to the balanced equation. For the given reaction 1 mole rxn would be the same as 1 mole of CH4(g) reacted, or 2 moles of O2(g) reacted, or 1 mole of CO2 formed, or 2 moles of H2O formed. Since the number of moles of each reactant and product are related by the balanced equations, knowing what is happening to one of them is the same as knowing all of them. We wrap that concept up in a single idea that is the "mole reaction."
To relate what actually happens in a real situation (extensive) to the theoretical idea (intensive) we need to be able to get from the reaction enthalpy (per mole reaction) to the actual enthalpy (the heat at constant pressure). To do this I need to multiply by the number of moles of "reaction."
\[q_p = \Delta H = n \times \Delta H_{\rm rxn}^\circ \]
where n is the number of "moles of reaction." For the above example, if I reacted 1 mole of O2 this would only be 0.5 "mole reaction" since I need 2 moles of O2 in the balanced equation.
While chemists are typically interested in changes in enthalpy there are times in which we would like to look at the change in internal energy instead. Most of the time these two are essentially equal. This is because most chemical changes involve minimal energy transfer in the form of work. This is not the case for chemical reactions that involve gas molecules where there is often a change in the volume at constant pressure.
To relate \(\Delta H\) and \(\Delta U\) for such reactions we need to think about the heat and the work at constant pressure.
\[\Delta U = q + w\]
That is the first law of thermodynamics. At constant pressure \(q = \Delta H\) and \(w = -P\Delta V\). Taking these two we see that
\[\Delta U = \Delta H - P\Delta V\]
If we are looking at a reaction at constant temperature and constant pressure, the only way the volume can change is if we have a change in the number of gas moles (assuming we have ideal gases). Thus we can rewrite the work term as
\[w = -P\Delta V = -\Delta n_{gas}RT\]
So the work can be found from the change in the number of moles of gas, \(\Delta n_{gas} = n_{\rm{gas\; final}}- n_{\rm{gas \;initial}}\). In these cases, we assume any volume change from solids or liquids is essentially zero since it will be much smaller than any volume changes for the gas. Using these assumptions if there is no change in the number of moles of gas, then \(\Delta H = \Delta U\).