Chemical equilibrium is an example of a dynamic equilibrium and not static equilibrium. Know the difference in the two. Static equilibrium is fixed and non-changing – like balancing weights on a balance beam. Dynamic equilibrium has no NET overall change but does have some given processes still proceeding. The process itself proceeds both forwards and backwards at exactly the same rate. Anything that you are constantly depleting via one process is simultaneously being replenished by another process. Stated chemically, equilibrium is achieved when the forward rate of reaction equals the reverse rate of reaction. That is a purely kinetic argument for equilibrium and we will study reaction kinetics (rates) in the second half of general chemistry (CH302). A complete understanding of equilibrium requires knowledge of both arguments (definitions) for the equilibrium state. This unit is about thermodynamics, which has our other definition of equilibrium. It is based purely on thermodynamic state functions. Lets get the thermodynamic argument for equilibrium established next.
The bottom line for the thermodynamic argument lies in the spontaneity of a reaction. The Second Law of Thermodynamics dictates what direction of change is the spontaneous direction. We know that indicator to be universal entropy (\(\Delta S_{\rm univ}\)), although in the previous section we showed how the free energy change (\(\Delta G\)) is an even better indicator for us because it is a purely system based state function. Once again, lets look at the definition of \(G\).
\[G = H - T\;S\]
You'll notice that \(G\) is made up from 3 other state functions. Also pay close attention to how \(G\) will change with \(T\). LOOK at the equation, as \(T\) increases, \(G\) must decrease. If we hold temperature (\(T\)) and pressure (\(P\)) constant, we get the following for the change in free energy:
\[\Delta G = \Delta H - T\Delta S\]
This powerful formula allows us to track spontaneity with a purely system state function, \(\Delta G\). It tracks via sign the opposite of the way that \(\Delta S_{\rm univ}\) does because \(\Delta G = -T\Delta S_{\rm univ}\). Now consider the 3 possible mathematical outcomes for \(\Delta G\):
\[\Delta G < 0\]
⊖ negative
spontaneous
\[\Delta G = 0\]
zero
equilibrium
\[\Delta G > 0\]
⊕ positive
non-spontaneous
We now have a new standard to judge spontaneity and equilibrium. ALL equilibrium processes must have a free energy change equal to zero. This is the same as saying that all the free energies (that’s plain ol’ \(G\) here) of all the reactants must equal the free energies of all the products – our “stalemate” condition for equilibrium.
Notice how \(\Delta G\)’s sign is controlled by the signs on \(\Delta H\) and \(\Delta S\). There are four distinct cases here. The following plot shows each of these cases:
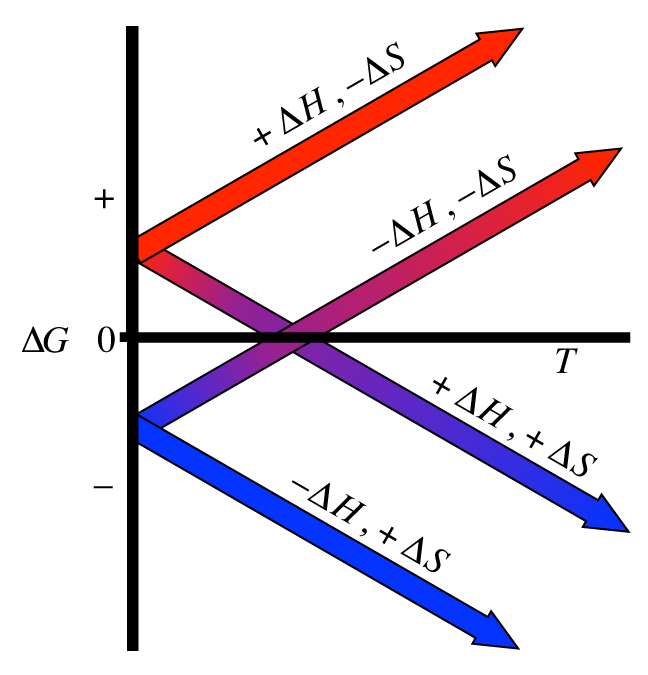
Note there are only 2 of those cases where equilibrium is even possible; those are the cases where the 2 plots cross the zero-point for \(\Delta G\). This is only when \(\Delta H\) and \(\Delta S\) have matching signs. Remember that we typically control the temperature of the reactions that we are running. Note how by controlling temperature, we, in effect, control the spontaneity as well.
Phase transitions occurring at their standard temperature (ice melting at 0 °C are reversible equilibrium processes. If you have an equilibrium process occurring, then \(\Delta G = 0\) and therefore at these temperatures
\(\displaystyle{\Delta H = T\Delta S}\) \(\displaystyle{\Delta S = {\Delta H \over T}}\) \(\displaystyle{T = {\Delta H \over \Delta S }}\)
For example, for water \(\Delta S_{fusion} = (\Delta H_{fusion} / 273.15 K)\) since the temperature for the phase transition is 0 °C (273.15 K). You can also use this idea to find the phase transition temperature if you know the enthalpy and the entropy changes.