The Aufbau Principle is the idea that we can find the electronic configuration of any element by simply "building up" from H and putting electrons into orbitals (wavefunctions) according to a rank in their energies. Aufbau means building up in German. Remember unlike in the hydrogen atom, the energy now depends both on \( n \) and \( \ell \). Generally as the \( \ell \) quantum number increases the energy is slightly higher. As a result 2s and 2p are no longer identical in energy in atoms with more than one electron. Now 2p is slightly higher than 2s. Moreover, as the energy levels get closer and closer together the ordering of the energies with respect to \( n \) can change. For example, we find that 3d is now higher in energy than 4s. Thankfully the general ranking of the energies of the subshells holds for most of the atoms. The order of the subshells is
\[1s<2s<2p<3s<3p<4s<3d<4p<5s<4d<5p\]
\[<6s<4f<5d<6p<7s<5f<6d<7p\]
We can determine the orbitals for the electrons in a multi-electron atoms by placing the electrons into subshells of ever increasing energy. We need to keep in mind that two electrons can go into each orbital. Thus a 3d subshell which has 5 d orbitals can "hold" 10 electrons. So we could place up to 10 electrons into the 3d subshell before needing to move on to the next highest energy subshell which is the 4p.
You should also get familiar with the periodic table as a "map" of the Aufbau filling order. As you "build up" the electrons in each atom, keep in mind just where you are on the periodic table. Here is a periodic table layout that is only showing the Aufbau filling order orbital sets. This is a far more useful diagram than any grid with diagonals marked on it.
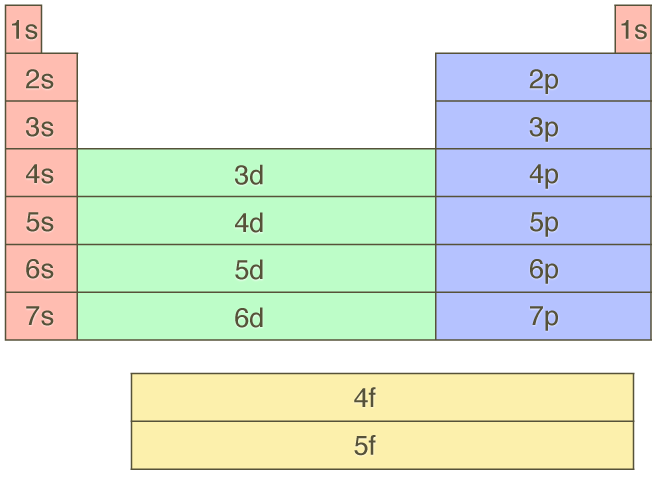
It is important to keep in mind that the Aufbau principle represents and approximate trend that holds in most cases. There are however exceptions to these rules. They will be covered in more detail later, but as a general idea the exception occur at places where a subshell can either be completely filled (or half-filled). Don't stress about the exceptions. Be very happy that this simple idea caputres 90% of the quantum mechanics of a very complicated problem. After this you can learn about the handful of exceptions and what they might tell us.